We know that our universe is a bit unreal. The stars we see in the night sky, for instance, are not really there. They may have moved or even died by the time we get to see them. It takes light time to travel from the distant stars and galaxies to reach us. We know of this delay. The sun that we see now is already eight minutes old by the time we see it, which is not a big deal. If we want to know what is going on at the sun right now, all we have to do is to wait for eight minutes. Nonetheless, we do have to “correct” for the delay in our perception due to the finite speed of light before we can trust what we see.
Now, this effect raises an interesting question — what is the “real” thing that we see? If seeing is believing, the stuff that we see should be the real thing. Then again, we know of the light travel time effect. So we should correct what we see before believing it. What then does “seeing” mean? When we say we see something, what do we really mean?
Seeing involves light, obviously. It is the finite (albeit very high) speed of light influences and distorts the way we see things, like the delay in seeing objects like stars. What is surprising (and seldom highlighted) is that when it comes to seeing moving objects, we cannot back-calculate the same way we take out the delay in seeing the sun. If we see a celestial body moving at an improbably high speed, we cannot figure out how fast and in what direction it is “really” moving without making further assumptions. One way of handling this difficulty is to ascribe the distortions in our perception to the fundamental properties of the arena of physics — space and time. Another course of action is to accept the disconnection between our perception and the underlying “reality” and deal with it in some way.
This disconnect between what we see and what is out there is not unknown to many philosophical schools of thought. Phenomenalism, for instance, holds the view that space and time are not objective realities. They are merely the medium of our perception. All the phenomena that happen in space and time are merely bundles of our perception. In other words, space and time are cognitive constructs arising from perception. Thus, all the physical properties that we ascribe to space and time can only apply to the phenomenal reality (the reality as we sense it). The noumenal reality (which holds the physical causes of our perception), by contrast, remains beyond our cognitive reach.
One, almost accidental, difficulty in redefining the effects of the finite speed of light as the properties of space and time is that any effect that we do understand gets instantly relegated to the realm of optical illusions. For instance, the eight-minute delay in seeing the sun, because we can readily understand it and disassociate it from our perception using simple arithmetic, is considered a mere optical illusion. However, the distortions in our perception of fast moving objects, although originating from the same source are considered a property of space and time because they are more complex. At some point, we have to come to terms with the fact that when it comes to seeing the universe, there is no such thing as an optical illusion, which is probably what Goethe pointed out when he said, “Optical illusion is optical truth.”
 The distinction (or lack thereof) between optical illusion and truth is one of the oldest debates in philosophy. After all, it is about the distinction between knowledge and reality. Knowledge is considered our view about something that, in reality, is “actually the case.” In other words, knowledge is a reflection, or a mental image of something external. In this picture, the external reality goes through a process of becoming our knowledge, which includes perception, cognitive activities, and the exercise of pure reason. This is the picture that physics has come to accept. While acknowledging that our perception may be imperfect, physics assumes that we can get closer and closer to the external reality through increasingly finer experimentation, and, more importantly, through better theorization. The Special and General Theories of Relativity are examples of brilliant applications of this view of reality where simple physical principles are relentlessly pursued using the formidable machine of pure reason to their logically inevitable conclusions.
The distinction (or lack thereof) between optical illusion and truth is one of the oldest debates in philosophy. After all, it is about the distinction between knowledge and reality. Knowledge is considered our view about something that, in reality, is “actually the case.” In other words, knowledge is a reflection, or a mental image of something external. In this picture, the external reality goes through a process of becoming our knowledge, which includes perception, cognitive activities, and the exercise of pure reason. This is the picture that physics has come to accept. While acknowledging that our perception may be imperfect, physics assumes that we can get closer and closer to the external reality through increasingly finer experimentation, and, more importantly, through better theorization. The Special and General Theories of Relativity are examples of brilliant applications of this view of reality where simple physical principles are relentlessly pursued using the formidable machine of pure reason to their logically inevitable conclusions.
But there is another, competing view of knowledge and reality that has been around for a long time. This is the view that regards perceived reality as an internal cognitive representation of our sensory inputs. In this view, knowledge and perceived reality are both internal cognitive constructs, although we have come to think of them as separate. What is external is not the reality as we perceive it, but an unknowable entity giving rise to the physical causes behind sensory inputs. In this school of thought, we build our reality in two, often overlapping, steps. The first step consists of the process of sensing, and the second one is that of cognitive and logical reasoning. We can apply this view of reality and knowledge to science, but in order do so, we have to guess the nature of the absolute reality, unknowable as it is.
The ramifications of these two different philosophical stances described above are tremendous. Since modern physics has embraced a non-phenomenalistic view of space and time, it finds itself at odds with that branch of philosophy. This chasm between philosophy and physics has grown to such a degree that the Nobel prize winning physicist, Steven Weinberg, wondered (in his book “Dreams of a Final Theory”) why the contribution from philosophy to physics have been so surprisingly small. It also prompts philosophers to make statements like, “Whether ‘noumenal reality causes phenomenal reality’ or whether ‘noumenal reality is independent of our sensing it’ or whether ‘we sense noumenal reality,’ the problem remains that the concept of noumenal reality is a totally redundant concept for the analysis of science.”
From the perspective of cognitive neuroscience, everything we see, sense, feel and think is the result of the neuronal interconnections in our brain and the tiny electrical signals in them. This view must be right. What else is there? All our thoughts and worries, knowledge and beliefs, ego and reality, life and death — everything is merely neuronal firings in the one and half kilograms of gooey, grey material that we call our brain. There is nothing else. Nothing!
In fact, this view of reality in neuroscience is an exact echo of phenomenalism, which considers everything a bundle of perception or mental constructs. Space and time are also cognitive constructs in our brain, like everything else. They are mental pictures our brains concoct out of the sensory inputs that our senses receive. Generated from our sensory perception and fabricated by our cognitive process, the space-time continuum is the arena of physics. Of all our senses, sight is by far the dominant one. The sensory input to sight is light. In a space created by the brain out of the light falling on our retinas (or on the photo sensors of the Hubble telescope), is it a surprise that nothing can travel faster than light?
This philosophical stance is the basis of my book, The Unreal Universe, which explores the common threads binding physics and philosophy. Such philosophical musings usually get a bad rap from us physicists. To physicists, philosophy is an entirely different field, another silo of knowledge, which holds no relevance to their endeavors. We need to change this belief and appreciate the overlap among different knowledge silos. It is in this overlap that we can expect to find great breakthroughs in human thought.
The twist to this story of light and reality is that we seem to have known all this for a long time. Classical philosophical schools seem to have thought along lines very similar to Einstein’s reasonings. The role of light in creating our reality or universe is at the heart of Western religious thinking. A universe devoid of light is not simply a world where you have switched off the lights. It is indeed a universe devoid of itself, a universe that doesn’t exist. It is in this context that we have to understand the wisdom behind the statement that “the earth was without form, and void” until God caused light to be, by saying “Let there be light.”
The Quran also says, “Allah is the light of the heavens and the earth,” which is mirrored in one of the ancient Hindu writings: “Lead me from darkness to light, lead me from the unreal to the real.” The role of light in taking us from the unreal void (the nothingness) to a reality was indeed understood for a long, long time. Is it possible that the ancient saints and prophets knew things that we are only now beginning to uncover with all our supposed advances in knowledge?
I know I may be rushing in where angels fear to tread, for reinterpreting the scriptures is a dangerous game. Such alien interpretations are seldom welcome in the theological circles. But I seek refuge in the fact that I am looking for concurrence in the metaphysical views of spiritual philosophies, without diminishing their mystical and theological value.
The parallels between the noumenal-phenomenal distinction in phenomenalism and the Brahman-Maya distinction in Advaita are hard to ignore. This time-tested wisdom on the nature of reality from the repertoire of spirituality is now being reinvented in modern neuroscience, which treats reality as a cognitive representation created by the brain. The brain uses the sensory inputs, memory, consciousness, and even language as ingredients in concocting our sense of reality. This view of reality, however, is something physics is yet to come to terms with. But to the extent that its arena (space and time) is a part of reality, physics is not immune to philosophy.
As we push the boundaries of our knowledge further and further, we are beginning to discover hitherto unsuspected and often surprising interconnections between different branches of human efforts. In the final analysis, how can the diverse domains of our knowledge be independent of each other when all our knowledge resides in our brain? Knowledge is a cognitive representation of our experiences. But then, so is reality; it is a cognitive representation of our sensory inputs. It is a fallacy to think that knowledge is our internal representation of an external reality, and therefore distinct from it. Knowledge and reality are both internal cognitive constructs, although we have come to think of them as separate.
Recognizing and making use of the interconnections among the different domains of human endeavor may be the catalyst for the next breakthrough in our collective wisdom that we have been waiting for.



 In order to make use of this principle, Einstein perhaps thought of it in pictures. What does acceleration mean? It is how fast the speed of something is changing. And what is speed? Think of something moving in a straight line — our cruising airplane, for instance, and call the line of flight the X-axis. We can visualize its speed by thinking of a time T-axis at right angles with the X-axis so that at time = 0, the airplane is at x = 0. At time t, it is at a point x = v.t, if it is moving with a speed v. So a line in the X-T plane (called the world line) represents the motion of the airplane. A faster airplane would have a shallower world line. An accelerating airplane, therefore, will have a curved world line, running from the slow world line to the fast one.
In order to make use of this principle, Einstein perhaps thought of it in pictures. What does acceleration mean? It is how fast the speed of something is changing. And what is speed? Think of something moving in a straight line — our cruising airplane, for instance, and call the line of flight the X-axis. We can visualize its speed by thinking of a time T-axis at right angles with the X-axis so that at time = 0, the airplane is at x = 0. At time t, it is at a point x = v.t, if it is moving with a speed v. So a line in the X-T plane (called the world line) represents the motion of the airplane. A faster airplane would have a shallower world line. An accelerating airplane, therefore, will have a curved world line, running from the slow world line to the fast one. The national newspaper of Singapore, the Straits Times, lauds the readable and conversation style used in The Unreal Universe and recommends it to anybody who wants to learn about life, the universe and everything.
The national newspaper of Singapore, the Straits Times, lauds the readable and conversation style used in The Unreal Universe and recommends it to anybody who wants to learn about life, the universe and everything.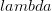 to observe the particle. The precision in the position we can hope to achieve is of the order of
to observe the particle. The precision in the position we can hope to achieve is of the order of 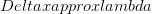 . In quantum mechanics, the momentum of each photon in the light beam is inversely proportional to the wavelength. At least one photon is reflected by the particle so that we can see it. So, by the classical conservation law, the momentum of the particle has to change by at least this amount(
. In quantum mechanics, the momentum of each photon in the light beam is inversely proportional to the wavelength. At least one photon is reflected by the particle so that we can see it. So, by the classical conservation law, the momentum of the particle has to change by at least this amount(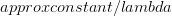 ) from what it was before the measurement. Thus, through perceptual arguments, we get something similar to the Heisenberg uncertainty principle
) from what it was before the measurement. Thus, through perceptual arguments, we get something similar to the Heisenberg uncertainty principle 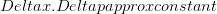
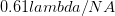 , where
, where 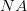 is the numerical aperture, which has a maximum value of one. Thus, the best spatial resolution is
is the numerical aperture, which has a maximum value of one. Thus, the best spatial resolution is 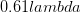 . Each photon in the light beam has a momentum
. Each photon in the light beam has a momentum 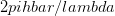 , which is the uncertainty in the particle momentum. So we get
, which is the uncertainty in the particle momentum. So we get 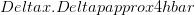 , approximately an order of magnitude bigger than the quantum mechanical limit.
, approximately an order of magnitude bigger than the quantum mechanical limit.